
Musicians create patterns of sounds in five dimensions:
A theory of music is an effort to describe the characteristic patterns of pitch and rhythm, the use of dynamics, the choices of instruments, and the spatial practices that describe a particular style of music.
Musical perception is most sensitive with respect to frequency and duration. Therefore, most theories of music are preoccupied with these two elements.
Musical patterns of frequency lend themselves particularly well to description from a physics perspective, and will therefore be a significant subject of study in this course. Musical patterns of duration (i.e., rhythm) are best understood in the context of psychological studies of the mechanisms for perceiving time, and will therefore not receive a great deal of attention in this course.
The basic pitch structure in music theory is an interval—a pair of frequencies presented melodically (i.e., successively) or harmonically (i.e., simultaneously).
Pythagoras and other early music theorists recognized that some intervals are especially useful in music. The ratios of these pairs of frequencies can be reduced to small, whole-number fractions:
By international agreement (adopted by the International Standards Organization (the ISO) in 1939 in London), the reference frequency for tuning instruments is 440 Hz.
A tone with a frequency of 440 Hz is called the note "A." A tone with a frequency an octave higher (i.e., 880 Hz), or any number of octaves higher, or an octave lower (i.e., 220 Hz), or any number of octaves lower is also called "A." This notion, that any tones an octave apart (or two or more octaves apart, for that matter) have the same note name and have virtually the same melodic and harmonic function, is called the principle of octave equivalence. It can be demonstrated that by transposing a melody an octave or more away from its harmonic accompaniment, for instance, the essential tonal relationships are maintained. Such is not the case if the melody is transposed by some interval other than an octave.
To determine musical pitches other than "A," it is necessary to apply a ratio other than 2:1, then. For Pythagoras, that ratio was 3:2—the ratio of the perfect fifth. Several additional musically useful frequencies can be determined this way. The objective is to assemble a sequence of musically useful pitches that correspond to the pitches that musicians use in melodies and the chords that accompany them.
Now arrange these frequencies in ascending order:
A-440, "B" (495 Hz), "D" (586.66 Hz), "E" (660 Hz), "G" (782.21 Hz), "A" (880 Hz)
And notate them, beginning with a blank staff of 5 parallel lines:

Establish a point of reference on the staff by marking one of the lines with the letter G, F, or C, called a clef:

(For further information about the history of musical notation, click here.)
And now place on the staff the notes that represent the frequencies calculated earlier:
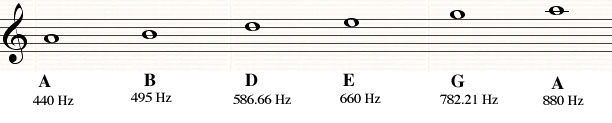
This arrangement of pitches, in which the frequencies used in a piece of music are arranged in ascending order within the span of an octave, is called a scale. The intervals between adjacent pitches in a scale are called steps. These steps can be arranged in ascending or descending order, as with a staircase or an escalator.
The particular set of pitches represented above as a scale form one of a set of universally useful scales called the pentatonic scales—literally, "5-toned" scales (excluding the octave duplication of the beginning note of the scale).
For an alternative representation of these five notes, placed across two octaves, click here.
Using the Pythagorean method shown above, here is another pentatonic scale:
Now arrange these frequencies in ascending order:
"C" (260.74 Hz), "D" (293.333 Hz), "E" (330 Hz), "G" (391.111 Hz), A-440, "C" (521.48 Hz)
And notate them:
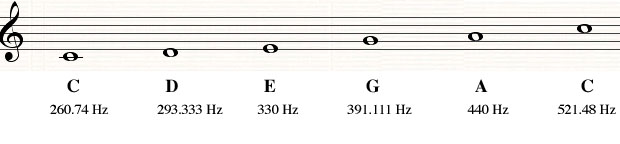
This form of the pentatonic scale can be found employed in many familiar melodies, such as "Amazing Grace" and the theme music for the television series "All in the Family." The use of pentatonic scales can be recognized in the music of a diversity of the world's cultures:
Even the blues scale is based on a pentatonic scale. Many music theorists regard the pentatonic scales as a set of "Ur" scales, from which most scales used in more recent times have evolved.
Pentatonic scales are characterized by gaps. For instance, the pentatonic scale above that begins and ends on "C" does not include an "F" or a "B." As the pentatonic scales evolved into more modern scales, these gaps became filled in with new notes.
In western music, these additional notes began to appear by the 11th century. Guido d'Arezzo, a priest and music theorist, codified a system for teaching melodies to singers that recognized the significance of the first of these additional notes.
Guido's system is based on a six-note scale, called a hexachord, that was arranged in the symmetrical pattern of two successive whole steps (i.e., major seconds, with a frequency ratio of 9:8 or something close to this), followed by a half step (i.e., a minor second, with a frequency ratio of 16:15 or something like this), followed by two more consecutive whole steps: W — W — H — W — W.
To these six notes, Guido assigned the following syllables (taken from what was then a familiar chant called "Ut queant laxis"): ut, re, mi, fa, sol, la. The interval between "mi" and "fa" is the half step. These syllables continue to be used by music students today.
There were three forms of the hexachord:
No new notes were necessary to notate the natural or the hard hexachords:
| NATURAL | ut | (W) | re | (W) | mi | (H) | fa | (W) | sol | (W) | la |
| C | D | E | F | G | A |
| HARD | ut | (W) | re | (W) | mi | (H) | fa | (W) | sol | (W) | la |
| G | A | B | C | D | E |
However, for the pattern of whole steps and half steps (W — W — H — W — W ) to be maintained for the soft hexachord, it was necessary to introduce a new note, a half-step lower than "B." This became known as "round B" or, as it is now known, "B-flat:"
| SOFT | ut | (W) | re | (W) | mi | (H) | fa | (W) | sol | (W) | la |
| F | G | A | B-flat | C | D |
By similar processes, additional notes (such as F-sharp) were subsequently introduced. This is the origin of the notes now played by the black keys of the piano.
Within a few centuries, the hexachords described by Guide d"Arezzo had evolved into seven-toned "modal" scales (eight tones, if you count the octave duplication at the end of the scale of the note that serves as the first note). In these so-called "modal" scales, all of the notes of the musical alphabet are represented, and all of the consecutive intervals are major or minor seconds—i.e., whole steps or half steps. One of these scale patterns, by virtue of its heavy use, has come to be known to theorists of Western music as the "major" scale:

The general part of the name of an interval (e.g., the "octave" in "perfect octave" or the "second" in "minor second") can be determined by counting the number of lines and spaces on the staff that are used to notate the interval:
The specific part of the name of an interval (e.g., the "perfect" in "perfect octave" or the "major" in "major third") can be determined by reference to the major scale formed on the lower note of the interval. A major scale has this pattern of whole steps and half-steps: W — W — H — W — W — W — H.
If the top note of the interval occurs in the major scale formed on the bottom note of the interval:
Seconds, thirds, sixths, and sevenths that are a half-step smaller than the ones that occur in the major scale are "minor."
Seconds, thirds, sixths, and sevenths that are a half-step larger than the ones that occur in the major scale are "augmented."
Fourths, fifths, and octaves that are a half-step smaller than the ones that occur in the major scale are "diminished."
Fourths, fifths, and octaves that are a half-step larger than the ones that occur in the major scale are "augmented."
An augmented fourth consists of three whole steps, and is called a tritone. It is a difficult interval to sing and was regarded in the Middle Ages as "diabolus en musica" (the Devil in music). It was "avoided like the Plague" by singers and theorists alike at that time, but has since found a valued place in the blues, jazz, and other musical styles.
As the specific sizes of intervals are altered on the staff it becomes necessary to add or remove sharp or flat signs from the notes.
A chord is a combination of intervals. An analysis by a music theorist of the intervals most frequently used in chords would show that the perfect octave, perfect fifth, perfect fourth (in some contexts), major third, and minor third are favored. With each of these intervals, the simultaneous sounding of frequencies is regarded by many listeners as pleasant. These intervals are identified as consonant intervals, and they combine well to form chords (aka: harmonies). Two chords of particular interest are:
The second (major or minor) is an interval that many listeners do not regard as pleasant when the pair of frequencies in the interval are presented simultaneously. Major and minor seconds are among those identified as dissonant intervals.
However, an analysis by a music theorist of the intervals used most frequently in melodies would show that seconds (aka, "steps") predominate. (The dissonance is mitigated by the fact that the intervals are not presented simultaneously ("harmonically") but are presented successively ("melodically") instead.).
See the textbook for information regarding the notation of rhythm and dynamics.
For additional information, see John Clough and Joyce Conley, Scales, Intervals, Keys, Triads, Rhythm and Meter, W. W. Norton.
prepared by S. Pellman, September 2002
updated September 2003